Program Summary:
| 13:30-14:30 | Shusen Yan, Central China Normal University |
| 14:30-15:30 | Zhaoli Liu, Capital Normal University |
| 15:30-16:00 | Tea Break |
| 16:00-17:00 | Changfeng Gui, University of Texas at San Antonio |
Talk Information:
Talk 1:
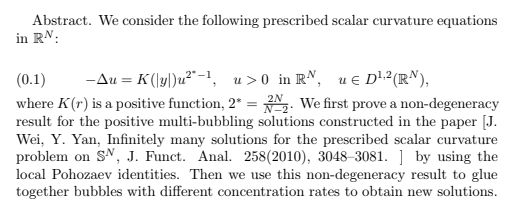
Non-Degeneracy of Multi-Bubbling Solutions for the Prescribed Scalar Curvature Equations and Applications
1:30-2:30 pm, November 14
by Shusen Yan, Central China Normal University
Abstract:
Biography:
Shusen Yan is a professor in the School of Mathematics and Statistics, Central China Normal University. He obtained his PhD in 1990 from the Institute of System Science, Chinese Academy of Science. He is well-known by his research work in nonlinear elliptic partial differential equations. In the last two decades, he mainly works on peak/bubbling solutions for nonlinear elliptic problems and successfully solves some important problems such as the estimates of the number of solutions for Ambrosetti-Prodi type elliptic problems which were raised in 1980s by Lazer and McKenna; the existence of infinitely many positive solutions for some non-compact elliptic problems, the exact number of solutions for Chern-Simons equations, the existence and local uniqueness of solutions for vortex patch problems from fluid dynamics.
Talk 2:
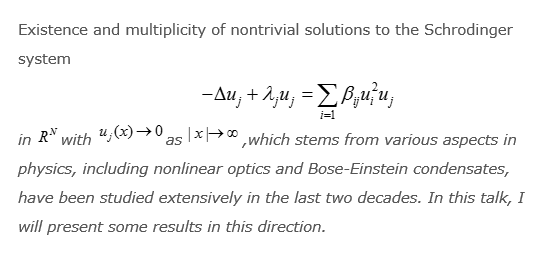
A Schrodinger System from Nonlinear Optics and Bose–Einstein Condensation
2:30-3:30 pm, November 14
by Zhaoli Liu, Capital Normal University
Abstract:
Biography:
Zhaoli Liu is a chair professor at Capital Normal University, a recipient of the National Science Fund for Distinguished Young Scholars, and a chair professor of the Changjiang Scholars Program. His research focuses on nonlinear analysis, invariant sets of descending flow, the critical points theory and their applications, and has obtained many important results on the existence and multiplicity, sign-changing solutions for elliptic equations.
Talk 3:
Axially Symmetric Solutions of Allen-Cahn Equation
4:00-5:00 pm, November 14
by Changfeng Gui, University of Texas at San Antonio
Abstract:
In this talk, I will present recent results on axially symmetric solutions of Allen-Cahn equation.
For the existence results, we show in three dimensional Euclidean space the existence of a complete family of axially symmetric solutions with a range of logarithmic growth rates, which may be regarded as the analogue of the family of catenoids and hence called two-end solutions. Nonexistence of two-end solution with a small growth rate is also shown, which differs from the theory of minimal surfaces.
For the classification of axially symmetric solutions with finite morse index, we show in dimension three that such solutions have finitely many ends. Furthermore, the solution has exactly two ends if its Morse index equals 1. It is also shown that there does not exist such a solution in dimensions between 4 and 10.
Biography:
Professor Changfeng Gui is a Fellow of the American Mathematical Society who has received numerous awards, including the PIMS Research Prize from the Pacific Institute of Mathematical Sciences, the Andrew Aisensdadt Prize from the Centre de Recherches Mathematiques (CRM), the IEEE Best Paper Award, and the Joint Research Fund for Overseas Chinese Young Scientists of NNFC. Professor Gui’s research interests include nonlinear PDEs, applied mathematics and image processing. He research papers have been published in international leading mathematics journals, including top journals such as Annals of Mathematics and Inventiones Mathematicae. Recently, his work on “The Sphere Covering Inequality and Its Applications” with his collaborators, solves a known conjecture on the best constant for the Moser-Trudinger inequalities.
Event by the NYU-ECNU Institute of Mathematical Sciences at NYU Shanghai